
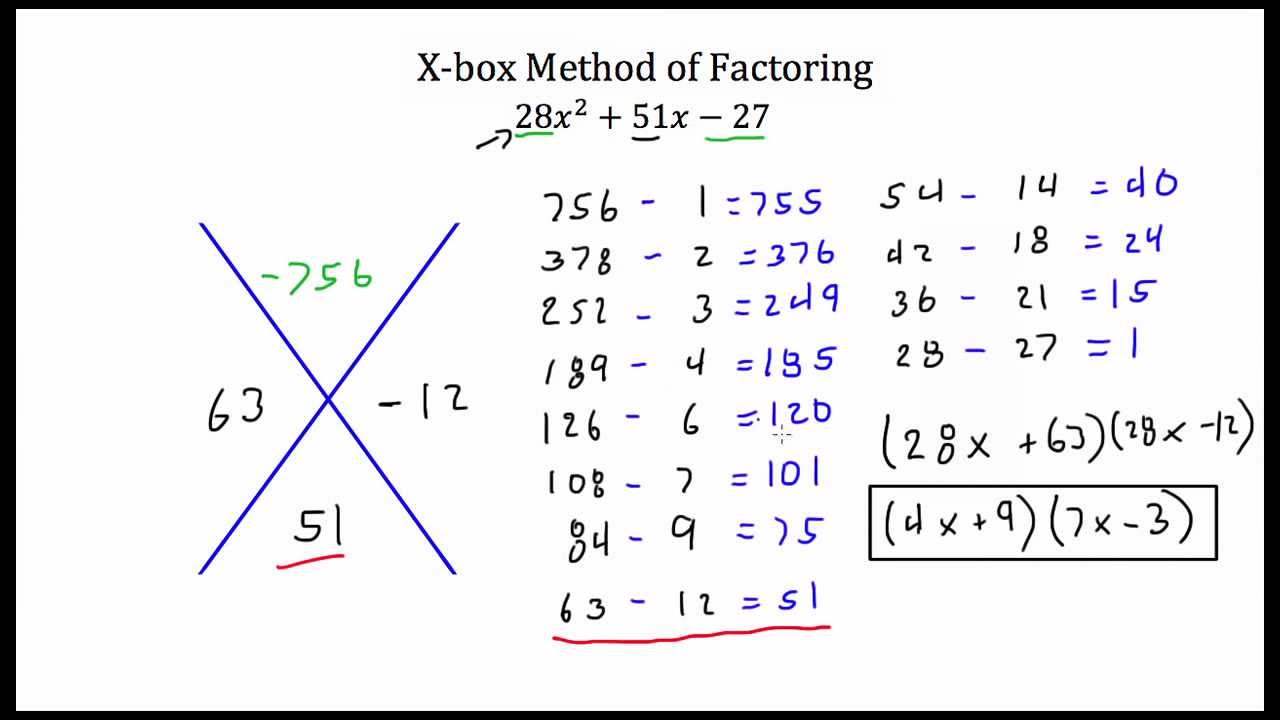
Note, however, that in general, this can be a fractional exponent.įor comparison, the inverse operation that would return the 5 from 5⁸ would be simply the ( 8-th) root. Symbolically, we can write the definition like so: 💡 logₐ(b) gives you the power to which you'd need to raise a in order to obtain b. In other words, it is a function that tells you the exponent needed to obtain the value. The logarithm (of base 5) would be the operation if we chose option 8. So what should the inverse operation give? If we have 5⁸, should it return 5 or 8? After all, we know that 5 + 8 = 8 + 5 and 5 * 8 = 8 * 5, but 5⁸ is very different from 8⁵. For exponents, however, the story gets more complicated. For multiplication, it's still pretty simple: it's division. Lucky for us, mathematics, and the whole world of science, other curious people found the answer.įor addition, it was easy: the inverse operation is subtraction. In this case, they wondered if there was a way to invert all these operations.
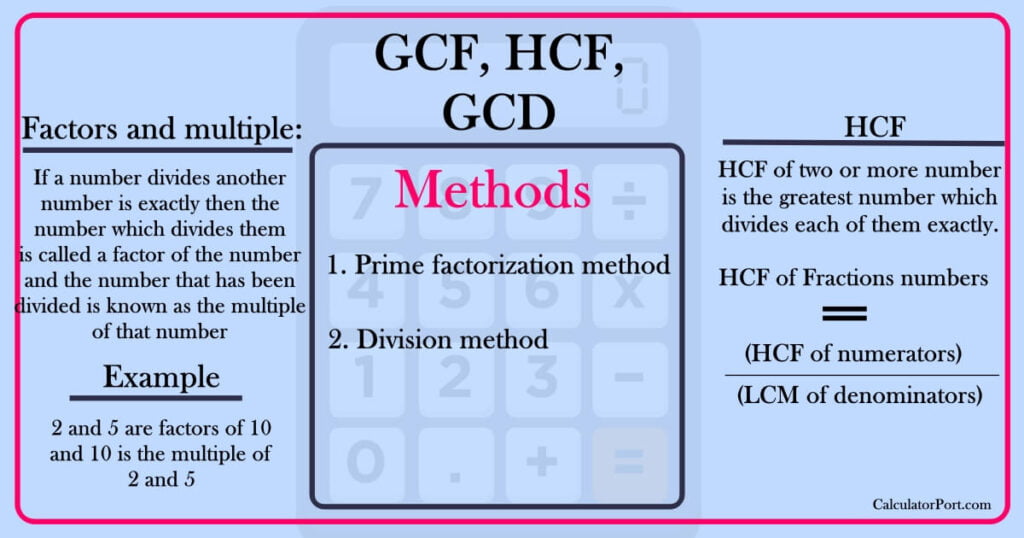
However, there is always that one curious person who asks the wildest questions. Then, an obvious question appeared: how could we write multiplying the same number several times? And again, there came some smart mathematician who introduced exponents. As soon as humanity learned to add numbers, it found a way to simplify the notation for adding the same number several times: multiplication.


 0 kommentar(er)
0 kommentar(er)
